Math can be divided into various subdivisions, with each branch playing a crucial role in solving real-life problems. Probability theory is one branch found in Mathematics. It entails quantifying uncertainty expected from the outcomes of an experiment that is conducted. Social Scientists, gamblers and statisticians are some of the professional that find the concepts of probability theory useful.
If you are tasked with a long syllabus, exams, tests, and homework, you might be tempted to look for probability help or statistics course help. Such assistance will work to your advantage in improving your overall grades and knowledge base. We have the right personnel to come to your rescue and get you back on track.
Definition of Probability
Probability refers to the likelihood that a certain event will happen. Probability of any event occurring lies in the range of between 0 and 1. If there is no way a certain event is likely to occur, then its probability equals zero. If one is sure that a certain event must happen, then its probability equals to one. The greater the probability, the more likely it is for that event to occur, and vice versa. Probability is calculated as the number of ways in which an event can happen, divided by the total number of outcomes
Example 1
If a coin is tossed once, one will either get head or tail. What is the probability of getting head?
P (E) = number of ways the event can happen/ total number of outcomes
Number of ways of getting heads=1
Total number of outcomes= 2
Therefore, P (H) =1/2= 0.5
Example 2
A tin box contains 9 black, 6 blue and 10 red marbles. If a marble is picked at random from the box, what is the probability that it is a red marble?
Red marbles = 10
Total marbles in box = 25
Therefore, P (red) = 10/25 = 0.4
Have you been struggling with tasks in Probability theory and need probability homework help? We understand that these concepts can prove difficult both for beginners and advanced students. Therefore, we have the most qualified experts to help you with any probability assignments while guaranteeing perfect grades.
What Are Experiments in Probability?
In math, an experiment is a process that will yield numeric data that can be used for analysis. Experiments are classified into deterministic and random experiments. These classifications are discussed below.
| Deterministic experiment | Random experiment |
|---|---|
| Results of this experiment remain the same irrespective of the number of times it is conducted | Results are subject to chance and therefore change with each experiment even if the conditions are unchanged |
| Example: length of a classroom remains the same each time it is measured | Example: rolling a die, tossing a coin, voting and so on |
Sample Spaces
In a random experiment, a sample space is the set of all possible outcomes in the experiment. A sample space can be viewed as a universal set in set theory. For example tossing a coin once would have, a sample space {H, T} while tossing two coins would have the sample space {HH, HT, TH, TT}. A sample point refers to the individual elements within the sample space
Do You Know That Getting Probability Help Services Can Improve Your Grades? If you have been experiencing poor grades or always submitting your work late, you should try our probability help services. With our writers and tutors, the concepts become easier to comprehend and the tasks completed on short notice. Therefore, if your probability homework is due urgently, you can have it done by a professional. Consequently, you will deliver high-quality work while observing the set deadlines.
Outcomes and Events
In probability, an outcome is the result of an experiment that s carried out under well-defined conditions. If a die is tossed, then any number between 1 and 6 is a probable outcome. On the other hand, an event is a subset of the sample space, comprising of a collection of the sample points.
Types of Events
Simple Event
If an event comprises of only one sample point within the sample space, it is known as a simple event. Therefore, there is only one outcome in this type of event
Compound Event
If an event comprises more than one sample point within the sample space, then it is a compound event. For instance, if you roll a die, the likelihood of even numbers includes the sample points {2, 4, 6)
Certain Event
An event that must occur is known as a certain event and its probability is equal to 1.
Impossible Event
This event can, under no circumstances, occur and therefore, has a probability of zero. For example, rolling a die and getting the number seven is an impossible event.
Equally Likely Events
These events have equal chances of occurring, like in the case of tossing a coin.
Mutually Exclusive Events
If certain events cannot occur at the same time, they are known as mutually exclusive events. When you toss a coin, you cannot get the head and tail at the same time.
Probability math help is one of the most common requests we receive from students. While some learners view this area as a walk in the park, to others it is the beginning of their downfall. However, this does not have to be the case since we have professional probability writers and tutors at your disposal. They can work on your homework, projects or even research papers to ensure that you maintain perfect scores in the class. Our tutors will walk you through the probability topics that are giving you sleepless nights until you fully grasp them.
Venn Diagrams
A Venn-Euler diagram is an instructive illustration representing the relationship between sets. A set refers to a collection of well-defined objects and it is denoted by capital letters. These objects present in the set are also known as elements. Venn diagrams can be used to calculate probability and they apply concepts of union, intersection, and complements. The Venn diagram below represents a set A, which is a subset of B meaning that all elements in A can be found in B.
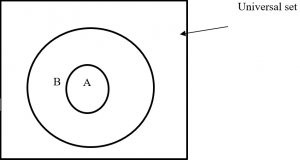
Probability and statistics go hand in hand, which makes it difficult to have one without the other. Consequently, the concepts taught become even more complex, warranting for probability, and statistics help. Fortunately, you can get such assistance from our writers affordably and conveniently. Each solution you receive is not only accurate, but also systematically calculated. We make it our business to ensure that students understand any piece of work they entrust us with.
Relationships between Sets and Axioms of Probability
Axioms of probability refer to the set of mathematical rules that probability must meet. If A and B are events and P (A) is the probability of event A and P (B) is the probability of event B,
Axiom 1: the axiom of positivity is 0≤P≤1. Therefore, negative probability does not exist, nor any that exceeds 1. For an event A, the probability of A not occurring can be represented as P (Ac) = 1-P (A). Also, P (A υ Ac) = P (S) = 1.
Axiom 2: the axiom of certainty means that P(S) =1 where S is the outcome space
Axiom 3: the axiom of sum implies that P (A) + P (B) = P (A+B) for any mutually exclusive events
In set theory, axiom 3 can be represented as P (A υ B) which is defined as the union of set A and set B.
Have you been seeking help with probability online and ending up more devastated than you began? A majority of students have had nasty experiences with academic writing help companies over quality, time of delivery, or prices. We assure you that our top-notch writers will deliver nothing but the best within the right time and at pocket-friendly charges.
Relative Frequency
A frequency refers to the number of times an event occurs within a given data set while relative frequency refers to the fraction of times an event occurs. The relative frequency is obtained by dividing each frequency by the total number in the sample space. It can be represented as a fraction, percent, or decimal number. The table below illustrates how to get the relative frequency.
| Data value | Frequency | Relative frequency |
|---|---|---|
| 1 | 4 | 4/20= 0.2 |
| 2 | 5 | 5/20= 0.25 |
| 3 | 2 | 2/20= 0.1 |
| 4 | 5 | 5/20= 0.25 |
| 5 | 4 | 4/20= 0.2 |
| Total | 20 | 1 |
Subjective Probability vs Objective Probability
| Subjective Probability | Objective Probability |
|---|---|
| Based on a person’s judgment or reasoning | Uses recorded observations to estimate the likelihood of an event occurring |
| No formal calculations since it is based on personal knowledge | Calculations are based on experiments, mathematical measurements, and statistics |
| Example: supporting a certain team during sports | Example: making financial decisions based on past statistical trends |
Looking for Statistics Probability Help? We Got You. We avail probability statistics help to students of any academic level at affordable prices. Our experienced writers and tutors go out of their way to ensure that each customer who uses our services is satisfied. We have been receiving overwhelming reviews from our esteemed customers, a reminder that we can only get better.